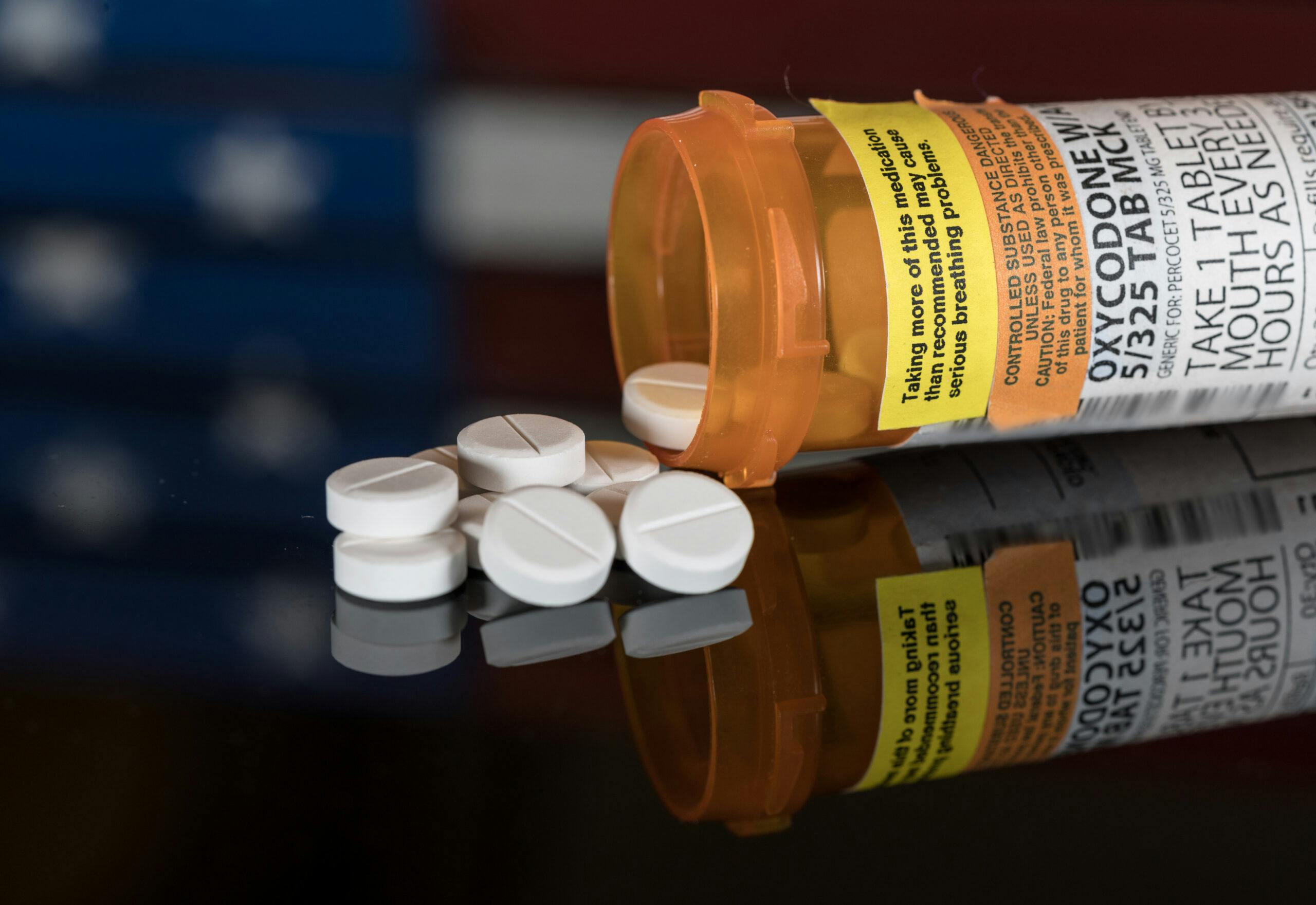



Vai trò của vật lý trị liệu và phục hồi chức năng đối với người cần chăm sóc
In Stock
$34.99
$29.99
Shipping and Returns Policy
- Deliver to United States » Shipping Policy «
- - Shipping Cost: $5.99
- - Handling time: 2-3 business days
- - Transit time: 7-10 business days
- Eligible for » Returns & Refund Policy « within 30 days from the date of delivery
Find similar items here:
a/an is a person who needs others to take care of
- NhữngConsider the function $f(x) = x^2 + 2x - 3$. What is the range of this function when the domain is restricted to $x \ge -2$? The function is given by $f(x) = x^2 + 2x - 3$. This is a quadratic function, and its graph is a parabola opening upwards because the coefficient of the $x^2$ term is positive (1 > 0). To find the vertex of the parabola, we can use the formula for the x-coordinate of the vertex $x_v = -\frac{b}{2a}$. In this function, $a = 1$ and $b = 2$, so $x_v = -\frac{2}{2(1)} = -\frac{2}{2} = -1$. Now, we can find the y-coordinate of the vertex by substituting $x_v$ back into the function $y_v = f(-1) = (-1)^2 + 2(-1) - 3 = 1 - 2 - 3 = -4$. So, the vertex of the parabola is at the point $(-1, -4)$. The domain of the function is restricted to $x \ge -2$. Since the parabola opens upwards, the minimum value of the function will occur at the vertex if the x-coordinate of the vertex is within the given domain. In this case, $x_v = -1$, and since $-1 \ge -2$, the vertex is within the restricted domain. The minimum value of the function within the restricted domain is the y-coordinate of the vertex, which is $-4$. Now we need to consider the behavior of the function as $x$ increases within the domain $x \ge -2$. Since the parabola opens upwards and the vertex is at $x = -1$, the function will increase for values of $x$ greater than $-1$. To find the upper bound of the range (if any), we need to examine the behavior of the function as $x$ goes towards infinity within the restricted domain. As $x \to \infty$, $f(x) = x^2 + 2x - 3 \to \infty$. Alternatively, we can evaluate the function at the boundary of the restricted domain, $x = -2$ $f(-2) = (-2)^2 + 2(-2) - 3 = 4 - 4 - 3 = -3$. Since the minimum value of the function within the domain $x \ge -2$ is $-4$ (at $x = -1$) and the function increases for $x > -1$, the range of the function for $x \ge -2$ will be all values greater than or equal to the minimum value. Therefore, the range of the function $f(x) = x^2 + 2x - 3$ when the domain is restricted to $x \ge -2$ is $[-4, \infty)$. Final Answer The final answer is $\boxed{[-4, \infty)}$
- Làm thế nào để giải quyết các tranh chấp pháp lý liên quan đến việc chăm sóc
- Những câu chuyện cảm động về tình yêu thương và sự hy sinh trong việc chăm sóc người thân
- Những thay đổi trong luật pháp Việt Nam liên quan đến quyền lợi của người cần chăm sóc
- Làm thế nào để vượt qua cảm giác cô đơn và bất lực khi chăm sóc người cần chăm sóc
- Những xu hướng mới trong lĩnh vực chăm sóc sức khỏe và chăm sóc dài hạn trên thế giới
- Vai trò của tình nguyện viên trong việc hỗ trợ người cần chăm sóc là gì
- Những lưu ý về văn hóa trong việc chăm sóc người lớn tuổi tại Việt Nam
- Làm thế nào để lập kế hoạch chăm sóc toàn diện
- Tiêu chuẩn chất lượng cho các cơ sở chăm sóc người cần chăm sóc là gì
-
Next Day Delivery by USPS
Find out more
Order by 9pm (excludes Public holidays)
$11.99
-
Express Delivery - 48 Hours
Find out more
Order by 9pm (excludes Public holidays)
$9.99
-
Standard Delivery $6.99 Find out more
Delivered within 3 - 7 days (excludes Public holidays).
-
Store Delivery $6.99 Find out more
Delivered to your chosen store within 3-7 days
Spend over $400 (excluding delivery charge) to get a $20 voucher to spend in-store -
International Delivery Find out more
International Delivery is available for this product. The cost and delivery time depend on the country.
You can now return your online order in a few easy steps. Select your preferred tracked returns service. We have print at home, paperless and collection options available.
You have 28 days to return your order from the date it’s delivered. Exclusions apply.
View our full Returns and Exchanges information.
Our extended Christmas returns policy runs from 28th October until 5th January 2025, all items purchased online during this time can be returned for a full refund.
No reviews yet. Only logged in customers who have purchased this product may leave a review.
